前言
PriorityQueue这个队列不知道大家使用过吗,反正我用的很少,主要对它不是很了解,今天我带领大家剖析下PriorityQueue这个优先级队列。
PriorityQueue介绍
顾名思义,PriorityQueue是优先队列的意思。优先队列的作用是能保证每次取出的元素都是队列中权值最小的。这里牵涉到了大小关系,元素大小的评判可以通过元素本身的自然顺序(natural ordering),也可以通过构造时传入的比较器。
- PriorityQueue实现了Queue接口,最大的特点是存取具有优先级,就是根据元素的顺序来决定
- PriorityQueue是一个无界的容器
- PriorityQueue底层是基于堆实现的
- 不允许放入null元素
- PriorityQueue不是线程安全的
 图片
图片
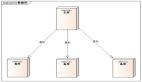
以上是PriorityQueue的类图,
- 继承了AbstractQueue抽象类,实现了Queue接口,具备队列的操作方法
- 实现了Seriablizable接口,支持序列化
构造方法
方法 | 说明 |
PriorityQueue() | 构造一个初始容量为11的优先队列 |
PriorityQueue(Comparator<? super E> comparator) | 构造一个自定义排序器的优先队列 |
PriorityQueue(SortedSet<? extends E> c) | 构造一个基于SortedSet内容的优先队列 |
关键方法
方法 | 说明 |
add(E e) | 添加元素,如果超过队列长度,抛出异常 |
offer(E e) | 添加元素,如果超过队列长度返回false |
remove() | 获取下个元素,如果没有抛出异常 |
poll() | 获取下个元素,如果没有返回null |
element() | 查看下个元素的内容,如果没有抛异常 |
peek() | 查看下个元素的内容,如果没有返回null |
使用案例
- 优先队列功能测试
@Test
public void test1() {
Queue<Integer> queue = new PriorityQueue<>();
queue.offer(5);
queue.offer(4);
queue.offer(1);
queue.offer(9);
queue.offer(3);
queue.offer(2);
// 打印,排序
Integer poll = null;
while ((poll = queue.poll()) != null) {
System.out.println(poll);
}
}运行结果:
 图片
图片
- 自定义排序器
@Test
public void test2() {
// 自定义排序,倒序
Queue<Integer> queue = new PriorityQueue<>(Collections.reverseOrder());
queue.offer(5);
queue.offer(4);
queue.offer(1);
queue.offer(9);
queue.offer(3);
queue.offer(2);
// 打印,排序
Integer poll = null;
while ((poll = queue.poll()) != null) {
System.out.println(poll);
}
}运行结果:
 图片
图片
实现机制
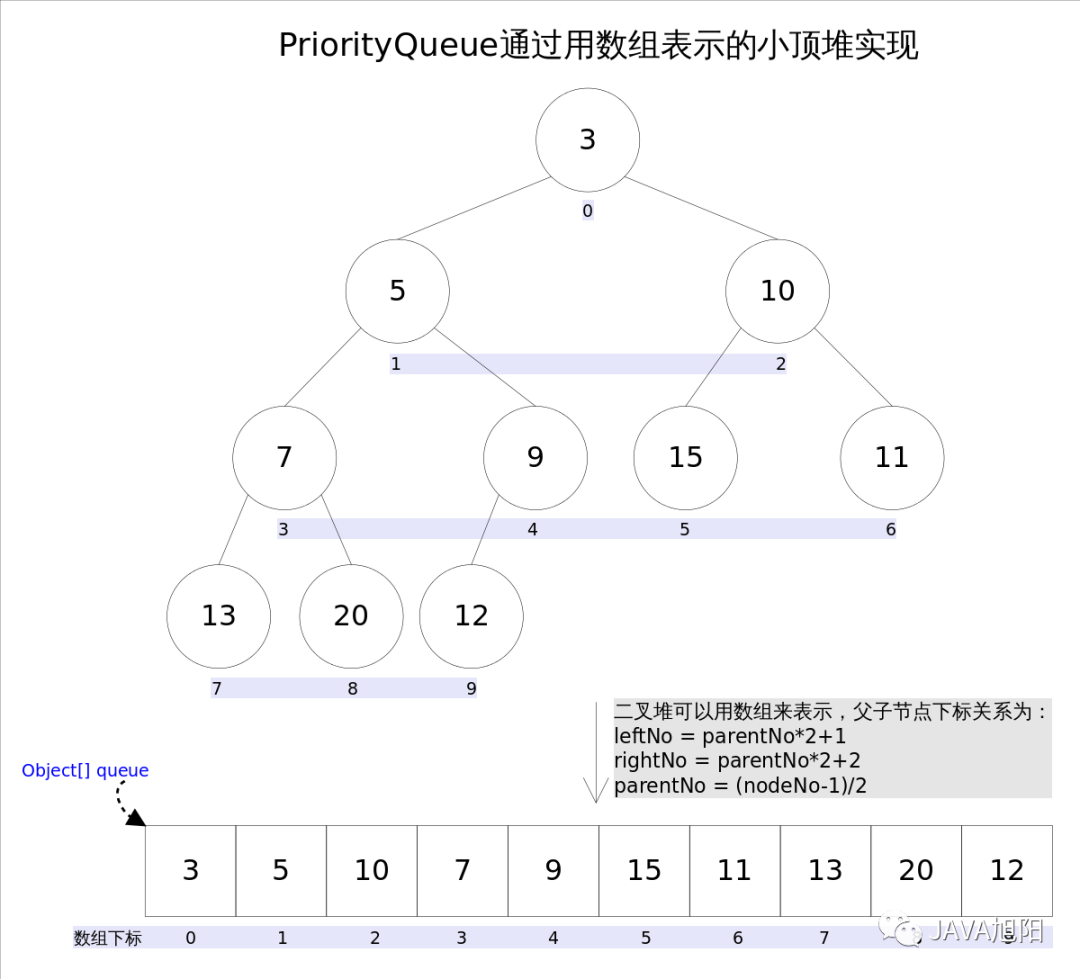
PriorityQueue通过堆实现,具体说是通过完全二叉树(complete binary tree)实现的小顶堆(任意一个非叶子节点的权值,都不大于其左右子节点的权值),也就意味着可以通过数组来作为PriorityQueue的底层实现。
 图片
图片
上图中我们给每个元素按照层序遍历的方式进行了编号,如果你足够细心,会发现父节点和子节点的编号是有联系的,更确切的说父子节点的编号之间有如下关系:
leftNo = parentNo*2+1
rightNo = parentNo*2+2
parentNo = (nodeNo-1)/2
通过上述三个公式,可以轻易计算出某个节点的父节点以及子节点的下标。这也就是为什么可以直接用数组来存储堆的原因。
源码解析
成员变量
transient Object[] queue;
/**
* The number of elements in the priority queue.
*/
private int size = 0;
/**
* The comparator, or null if priority queue uses elements'
* natural ordering.
*/
private final Comparator<? super E> comparator;
/**
* The number of times this priority queue has been
* <i>structurally modified</i>. See AbstractList for gory details.
*/
transient int modCount = 0;- queue就是实际存储元素的数组。
- size表示当前元素个数。
- comparator为比较器,可以为null。
- modCount记录修改次数。
构造方法
public PriorityQueue(int initialCapacity,
Comparator<? super E> comparator) {
// Note: This restriction of at least one is not actually needed,
// but continues for 1.5 compatibility
if (initialCapacity < 1)
throw new IllegalArgumentException();
this.queue = new Object[initialCapacity];
this.comparator = comparator;
}- 初始化了queue和comparator
添加元素offer
public boolean offer(E e) {
// 如果元素为空,抛出空指针
if (e == null)
throw new NullPointerException();
// 修改次数+1
modCount++;
int i = size;
// 首先确保数组长度是够的,如果不够,调用grow方法动态扩展。
if (i >= queue.length)
grow(i + 1);
size = i + 1;
// 如果是第一次添加,直接添加到第一个位置即可 (queue[0]=e)
if (i == 0)
queue[0] = e;
else
// 否则将其放入最后一个位置,但同时向上调整,直至满足堆的性质 (siftUp)
siftUp(i, e);
return true;
}private void grow(int minCapacity) {
int oldCapacity = queue.length;
// Double size if small; else grow by 50%
int newCapacity = oldCapacity + ((oldCapacity < 64) ?
(oldCapacity + 2) :
(oldCapacity >> 1));
// overflow-conscious code
if (newCapacity - MAX_ARRAY_SIZE > 0)
newCapacity = hugeCapacity(minCapacity);
queue = Arrays.copyOf(queue, newCapacity);
}如果原长度比较小,大概就是扩展为两倍,否则就是增加50%,使用Arrays.copyOf方法拷贝数组。
private void siftUp(int k, E x) {
// 如果比较器为空
if (comparator != null)
siftUpUsingComparator(k, x);
else
siftUpComparable(k, x);
}private void siftUpUsingComparator(int k, E x) {
while (k > 0) {
int parent = (k - 1) >>> 1;
Object e = queue[parent];
if (comparator.compare(x, (E) e) >= 0)
break;
queue[k] = e;
k = parent;
}
queue[k] = x;
}参数k表示插入位置,x表示新元素。k初始等于数组大小,即在最后一个位置插入。代码的主要部分是:往上寻找x真正应该插入的位置,这个位置用k表示。
怎么找呢?新元素(x)不断与父节点(e)比较,如果新元素(x)大于等于父节点(e),则已满足堆的性质,退出循环,k就是新元素最终的位置,否则,将父节点往下移(queue[k]=e),继续向上寻找。
总结
优先级可以有相同的,内部元素不是完全有序的,如果遍历输出,除了第一个,其他没有特定顺序。查看头部元素的效率很高,为O(1),入队、出队效率比较高,为O(log2(N)),构建堆的效率为O(N)。根据值查找和删除元素的效率比较低,为O(N)。